-
This forum contains old posts that have been closed. New threads and replies may not be made here. Please navigate to the relevant forum to create a new thread or post a reply.
-
Welcome to Tamil Brahmins forums.
You are currently viewing our boards as a guest which gives you limited access to view most discussions and access our other features. By joining our Free Brahmin Community you will have access to post topics, communicate privately with other members (PM), respond to polls, upload content and access many other special features. Registration is fast, simple and absolutely free so please, join our community today!
If you have any problems with the registration process or your account login, please contact contact us.
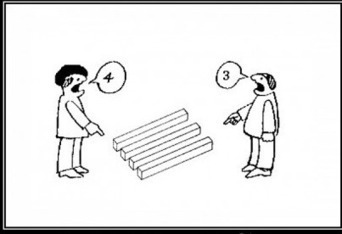
3 or 4 ?
- Thread starter P.J.
- Start date
- Status
- Not open for further replies.
Raji Ram
Active member
Mathematical proof is possible for the above drawing!
If the number of planks drawn in the left side is taken as 'n', the number of planks in the right side is 'n-1'
We need 2n+1 lines in total, to draw 'n' planks in the left side and three lines for EACH of the planks on the right.
The equation to find 'n' is:
2n +1 = 3 X (n-1) since we need 3 lines to represent a plank in the right side.
2n + 1 = 3n - 3
changing sides
3n - 2n = 1 + 3
Hence n = 4.
That is why four planks are shown in the left side and three on the right!

If the number of planks drawn in the left side is taken as 'n', the number of planks in the right side is 'n-1'
We need 2n+1 lines in total, to draw 'n' planks in the left side and three lines for EACH of the planks on the right.
The equation to find 'n' is:
2n +1 = 3 X (n-1) since we need 3 lines to represent a plank in the right side.
2n + 1 = 3n - 3
changing sides
3n - 2n = 1 + 3
Hence n = 4.
That is why four planks are shown in the left side and three on the right!
S
SANDHYAV
Guest
1. We need 2n+1 lines in total, to draw 'n' planks in the left side and three lines for EACH of the planks on the right.
The equation to find 'n' is:
2. 2n +1 = 3 X (n-1) since we need 3 lines to represent a plank in the right side.
1. how
2. why 3 times n-1
Raji Ram
Active member
1. We have taken 'n' as the number of planks in the LHS. Here, only the first plank needs three lines and the following have two more EACH.
1. We need 2n+1 lines in total, to draw 'n' planks in the left side and three lines for EACH of the planks on the right.
The equation to find 'n' is:
2. 2n +1 = 3 X (n-1) since we need 3 lines to represent a plank in the right side.
1. how
2. why 3 times n-1
Thus we need 3 + 2 + 2 and so on to draw in that manner. All the planks after the first need ONLY two lines. So total lines are 2n + 1 OK?
We have one plank LESS on the right hand side. Since the number of planks on LHS is denoted by 'n' the number on the RHS will be (n - 1)
Hope it is clear up to this.
2. We need three lines to denote EACH plank on the RHS. So, we have to multiply the number of planks in RHS by 3 to get the number of lines.
Since the lines drawn are the SAME for planks on LHS and RHS, we equate 2n + 1 and 3 x (n - 1) :thumb:
S
SANDHYAV
Guest
haha,
only horizontal lines !
also this 4-3 combination is possible only for 4-3 and not for 5-4
only horizontal lines !
also this 4-3 combination is possible only for 4-3 and not for 5-4
Raji Ram
Active member
YES! In fact ONLY for 4 and 3 if drawn in this way! :decision:haha,
only horizontal lines !
also this 4-3 combination is possible only for 4-3 and not for 5-4
prasad1
Active member
I agree with Rajiji,The drawing is wrong!
Check out the lines starting from the left side!
The 2nd z-axis line are wrong.
The 3rd Y-axis line is wrong.
P.J.
0
Are you not a math student P J Sir?
It is a very simple calculation.
Raji Madam
No...... I took only General Mathematics
Verala uttu kootra case illa , better than that !!
- Status
- Not open for further replies.
Latest ads
-
Free [Bride Wanted] Bride wantedBrahmin Telugu Niyogi Boy IT Oracle Bangalore Srinivasan aged 37
- Chinnu (+0 /0 /-0)
- Updated:
-
For rent [Property for Rent] Pallikkaranai spacious 3 bed room independent house3 bed room independent house with covered car parking
- jaradhva (+0 /0 /-0)
- Updated:
-
For rent [Property for Rent] Spacious independent house for rent at PallikkaranaiPallikkaranai independent house for rent Negotiable
- jaradhva (+0 /0 /-0)
- Updated:
- Expires
-
Wanted [Cook Wanted] Brahmin cook needed in porur chennaiBrahmin cook needed in Porur,chennai ,pl contact harims24@gmail.com Thankyou Hariharan
- Hari M S (+0 /0 /-0)
- Updated:
-
For sale [Property for Sale] 3-BHK Villa in seniors' community in CoimbatoreReady to move in villa for Sale in a premium gated retirement community near Kovaipudur, Coimbatore
- srikamal (+0 /0 /-0)
- Updated: